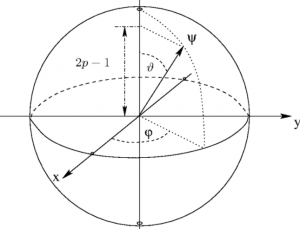
Qubit (Quantum Bit) is the fundamental unit of information in quantum computing as like bit which is the basic unit of information in classical computing. However, unlike bit in the classical computing which can exist in one of two states such as 0 and 1 at any given point of time, the qubit can be found to exist in states 0, 1 or the superposition state which, simply speaking, can be said to be both 0 and 1.
In this post, you will learn some of the following:
- Qubit and Superposition State
- Superposition state explained with examples
Qubit and Superposition State
The following are some of the important points about the state of Qubits:
- The qubit can be found to exist in the state of 0, 1 or the superposition state which represents the state of both 0 and 1.
- However, when measured, Qubit would found to be in only one of the two states 0 and 1 and not the superposition state. This is very important to understand.
The state in Quantum theory is represented using | and >. However, for the sake of understanding, we will use vector notation to represent the 0 and 1 state. Thus, the following holds true:
- The state of 0 can be represented using [latex]\vec{0}[/latex]
- The state of 1 can be represented using [latex]\vec{1}[/latex]
- The superposition state can be represented as the following:
[latex]\vec{\gamma}[/latex] = [latex]\alpha\vec{0} + \beta\vec{1}[/latex]where [latex]\alpha[/latex] and [latex]\beta[/latex] are complex numbers and [latex]\vec{\gamma}[/latex] is the superposition state of state [latex]\alpha\vec{0}[/latex] and [latex]\alpha\vec{1}[/latex]. Recall that the complex numbers are of the form, c = a + ib, where i = [latex]\sqrt{-1}[/latex].
As per the quantum mechanics, the modulus squared of [latex]\alpha[/latex] and [latex]\beta[/latex] represents the probability of finding the qubit in state [latex]\vec{0}[/latex] and [latex]\vec{1}[/latex] respectively.
The fact that probability must sum upto 1 leads to following equation which could be used for determining value of [latex]\alpha[/latex] and [latex]\beta[/latex].
[latex]|{\alpha}|^2 + |{\beta}|^2 = 1[/latex]
One needs to note that if [latex]{\alpha}, {\beta}[/latex] are real complex numbers with imaginary coefficients such as (x + iy), the way to find the modulus squared is to multiple the complex number with its conjugate. This esssentially means that modulus squared for complex number [latex]x + iy[/latex] is following:
[latex](x + iy)\times(x – iy)[/latex]
Superposition states explained with examples
Example 1: Let’s determine if the below can be said to represent a valid superposition state of a qubit:
[latex]\frac{1}{2}\vec{0} + \frac{\sqrt{3}}{2}\vec{1} [/latex]
The coefficient of [latex]\vec{0}[/latex] is the complex number, [latex]\frac{1}{2}[/latex]. The modulus squared of the coefficient, [latex]\frac{1}{4}[/latex], is the probability that state 0 will happen.
The coefficient of [latex]\vec{1}[/latex] is the complex number, [latex]\frac{\sqrt{3}}{2}[/latex]. The modulus squared of the coefficient, [latex]\frac{3}{4}[/latex] is the probability that state 0 will happen.
Going by above, the following gets evaluated:
[latex](\frac{1}{2})^2 + (\frac{\sqrt{3}}{2})^2[/latex]
[latex] = \frac{1}{4} + \frac{3}{4} = 1[/latex]
Thus, it could be said that [latex]\frac{1}{\sqrt{2}}\vec{0} + \frac{1}{\sqrt{2}}\vec{1} [/latex] is a valid superposition state.
Example 2: Let’s consider another example and check whether it is a valid superposition state:
[latex]\frac{1}{\sqrt{2}}\vec{0} + \frac{1}{2}\vec{1}[/latex]
The sum of modulus squared of coefficients (probability) of states should be equal to 1. Let’s check this out.
[latex](\frac{1}{\sqrt{2}})^2 + (\frac{1}{2})^2[/latex]
[latex] = \frac{1}{2} + \frac{1}{4} = \frac{3}{4} \ne 1[/latex]
Thus, the superposition state represented by above is not a valid superposition state.
Further Reading / References
- Quantum Superposition
- Book – Quantum Computing Explained (David McMahon). It is a great book for learning quantum computing. If you are planning to get deeper understanding of the book, you may not want to miss this one.
Summary
In this post, you learned about some of the following:
- Introduction to Superposition states of Qubit
- Superposition state explained with examples
Did you find this article useful? Do you have any questions about this article or understanding superposition states of Qubit in Quantum computing? Leave a comment and ask your questions and I shall do my best to address your queries.
Check out my latest book titled as First Principles Thinking: Building winning products using first principles thinking.
- Agentic Reasoning Design Patterns in AI: Examples - October 18, 2024
- LLMs for Adaptive Learning & Personalized Education - October 8, 2024
- Sparse Mixture of Experts (MoE) Models: Examples - October 6, 2024
I found it very helpful. However the differences are not too understandable for me